BQ #3: Unit T Concepts 1-3

How do the graphs of sine and cosine relate to each of the others?

1. Tangent
The ratio for tangent is sin/cos. So the asymptotes are located when tangent is 0, this would only happen when cos is equal to 0. Since cos is the x-value the asymptotes are located where x=0. That would be at pi/2 and 3pi/2.  If we recall from the unit circle all trig functions in the first quadrant are positive. Both sin and cos are pos. in the first quadrant so that means that  when you divide one by the other, you end up with a positive value for tangent. For quad.2 we know that sin is positive and cosine is neg. This means we get a negative value for tangent.in the third quad. sin and cos are both negative so we get a positive value for tan. In quad 4sin is pos and cos is neg. A and get a neg. value for tangent. The graph for tangent is pos, neg, pos, neg. When we graph it within its period and based on the location of the asymptotes it will be uphill or downhill.

2. Cotangent

The trig ratio for cotangent is cos/sin. So the asymptotes are located where sin is equal to 0(y). The asymptotes would be located at 0 and 180 because y=0 there.To find out how the appearance for cotangent graphs we can use the trig ratio for cotangent looks, we can use the  trig ratio for cot (cos/sin). Sin and cos are positive in the first quadrant, so when you divide one by the other, you end up with a positive value for cot. For quadrant 2 we get a negative value for cot. For the third quadrant we get a positive value for cot. In  the fourth quadrant 4,we get a negative value. The graph for cotangent is pos, neg, pos, neg. When we graph it within its period of 0 and pi, the graph will begin close to the asymptote above the x-axis.  When it enters second quad. it will be negative so we have to draw it downhill.

https://www.desmos.com/calculator/hjts26gwst

3. Secant

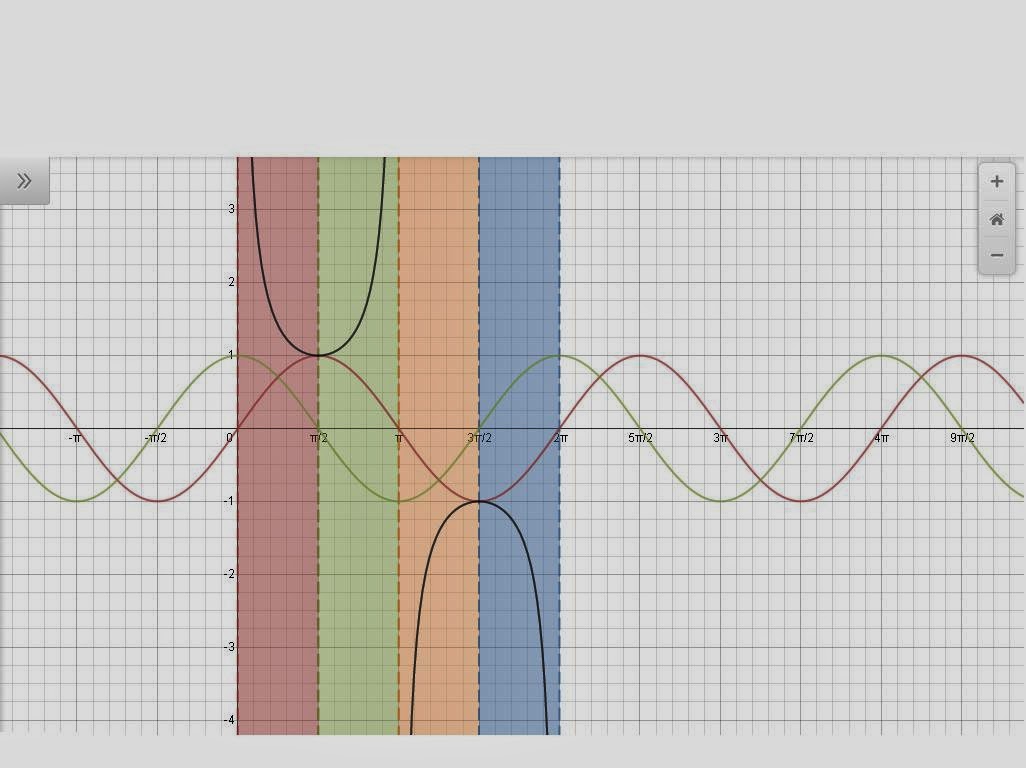
Secant is the reciprocal of cosine. It has a ratio of 1/cos. Since cosine is the denominator when it equals zero we will have asymptotes. Secant has asymptotes at pi/2 and 3pi/2(keep in mind that they go on forever to the left and right). These asymptotes are the only ones within the period. If secant is positive then the graph will go upward and if its negative the graph will go downward. Also if cosine is positive or negative secant will also.

https://www.desmos.com/calculator/hjts26gwst



 4.Cosecant?

 Cosecant is the reciprocal of sine. It has a ratio of 1/sin. We get asymptotes when the denominator equals zero. Cosecant has asymptotes at 0 and pi (they keep going).If sine is positive so will the cosecant graph. Cosecant is also affected by that.

https://www.desmos.com/calculator/hjts26gwst

BQ #4: Unit T Concept 3

Why is a "normal" tangent graph uphill, but a "normal" cotangent graph downhill?

          The normal tangent graph is uphill mainly because of the asymptotes. One of the reasons why its uphill is because of the asymptotes which are located at pi/2 and 3pi/2 (keep in mind they go on forever). If we recall from the unit circle the first quadrant was positive, the second negative, the third positive, and the fourth negative. So if we draw our asymptotes our graph has to be in between those asymptotes so that means where in the third and second quadrant. As mentioned above the third quadrant is positive and the second one is negative. So that means our graph has to be below the x-axis and then above the x-axis.

                       The normal cotangent graph is downhill because of its asymptotes.  The asymptotes for the normal cotangent graph are pi and 0. The labeling of the quadrants is the same. So it would be above the x- axis and then below as seen below.
    

BQ#5: Unit T: Concepts 1-3

Why do sines and cosine NOT have asymptotes, but the other four trig graphs do?
            

       To begin the ratio for sine is y/r and for cosine its x/r. If we recall r is 1 so that means that r can never equal 0 so we can't get an asymptote. We cannot get an asymptote because in order to get an asymptote we have to get an undefined and we can only get an undefined when we divide by zero.  Cosecant has a ratios of r/y and cotangent has a ratio of x/y. This means that we will get asymptotes when y=0. Also the asymptotes will be in the same place. The ratios for secant (r/x) and tangent (y/x) both end with x. So we get asymptotes when x=0. All trigs, except for sine and cosine, can be divide by zero this means that we can get an undefined and have asymptotes on the graph.

BQ #2: Unit T Concept Intro

1. How do the trig graphs relate to the Unit Circle?
      

 

 

 

          The trig graphs relate to the Unit Circle because it uses the negative and positive values of the quadrants in order to define how a period/graph will look  for a certain trig function. Each trig function forms a different looking graph. To begin the unit circle unfolds into a line. As we can see above the signs in parenthesis are the values that that quadrant would have on the unit circle (positive or negative). Since we unfolded the unit circle for the sine graph we are left with positive and on the left and negative on the right. This means that our graph will look like an uphill then a down hill. For the cosine we see an uphill, downhill, and then uphill again because of the positive, negative, negative, and positive value on the quadrants when we unfolded the Unit circle. Tangent/cotangent both have an uphill and downhill because they have a shorter period due to the positive and negative on the first two quadrants and positive negative in the third and fourth quadrant.

 

       -Period? - Why is the period for sine and cosine 2pi, whereas the period for tangent and cotangent is pi?

     The period for sine and cosine is 2pi because it takes four quadrants to repeat the same pattern over and over again. Tangent and cotangent has a period of pi because since it already has a positive and a negative value on the first two quadrants it only takes pi to repeat the pattern.

 

        -Amplitude? - How does the fact that sine and cosine have amplitudes of one (and the other trig functions don't have amplitudes) relate to what we know about the Unit Circle?

        The fact that sine and cosine have amplitudes of one relates to what we know about the unit circle because sine and cosine cannot be bigger that one. Since sine and cosine has a ratio of y/r and x/r and r =1. Sine and cosine can only be between -1 and 1.

Reflection#1: Unit Q Concepts 1 & 5

1. To begin proving an identity is very different from solving a problem. To prove an identity, you have to use identities to show that one side of the equation can be transformed into the other side of the equation. You don't plug values into the identity to prove anything. There are several different identities you can plug in. We also have to keep in mind that we can not touch the right side when verifying.

2. One of the main tricks that I found useful were trying to maintain sin and cos in the problem. Another trick that I found helpful was knowing when to combine and break up fractions so that the number of terms will match. The last trick that I found helpful was factoring out the GCF.

3.There are several ways that we can approach a problem. However, there are several things that we can do first such as looking for a GCF, substituting an identity, multiplying by the conjugate(when the denominator is a binomial), combine fractions with binomial denominators, and factoring.